, where ｙ１, ｙ2, …… ｙn are distribution of individual income of n samples, and f is an average value of them.
Therefore f is given as f = ( ｙ１ + ｙ2 + …… + ｙn) / n
Gini's coefficient is easily calculated with the use of Excel Application. This coefficient measures the degree of inequality in income of community. This value ranges between 0 to 1, where extreme zero value means an ideal equlity.
Gini's coefficient is defined as , where ｙ１, ｙ2, …… ｙn are distribution of individual income of n samples,
and f is an average value of them.
Therefore f is given as f = ( ｙ１ + ｙ2 + …… + ｙn) / n
The values ｙ１, ｙ2, …ｙn are filled in painted cells as demonstrated in the figure above.
C3 cell is typed as 『 =abs(c$2-$b3) 』 , and all cells in the table are copied entirely.
(note) Excel's ABS-function gives Absolute value, i.e. | 15 - 40 | equals to 25.
B8 cell is typed as『 =sum(c3:g7) 』 , indicating the
B9 is an average value of n samples i.e. 『 =average(b3:b7) 』 and B10 consists of 『 =count(b3:b7) 』, meaning the count of values corresponding the number of sample (n).
Gini's coefficient, B11 cell is calculated as 『 =b8/(2*b10*b10*b9) 』.
Description of B11 is also available as 『 =b8/(2*b10^2*b9) 』. 『 ^2 』 covers a value of number square, and 『 ^3 』 covers that of cubic. You can get a similar result if you copy the subsequent table and paste on your Excel Sheet. The item "sample" must be set in A1 cell.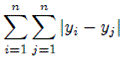
value in the Gini's definition.
| sample | ||||||
| 15 | 25 | 40 | 80 | 180 | ||
| 15 | =ABS(C$2-$B3) | =ABS(D$2-$B3) | =ABS(E$2-$B3) | =ABS(F$2-$B3) | =ABS(G$2-$B3) | |
| 25 | =ABS(C$2-$B4) | =ABS(D$2-$B4) | =ABS(E$2-$B4) | =ABS(F$2-$B4) | =ABS(G$2-$B4) | |
| 40 | =ABS(C$2-$B5) | =ABS(D$2-$B5) | =ABS(E$2-$B5) | =ABS(F$2-$B5) | =ABS(G$2-$B5) | |
| 80 | =ABS(C$2-$B6) | =ABS(D$2-$B6) | =ABS(E$2-$B6) | =ABS(F$2-$B6) | =ABS(G$2-$B6) | |
| 180 | =ABS(C$2-$B7) | =ABS(D$2-$B7) | =ABS(E$2-$B7) | =ABS(F$2-$B7) | =ABS(G$2-$B7) | |
| total amount | =SUM(C3:G7) | |||||
| average (f) | =AVERAGE(B3:B7) | |||||
| samples (n) | =COUNT(B3:B7) | |||||
| Gini's coeff.（ｇ） | =B8/(2*B10*B10*B9) |
